1. Home
  2. Research
  3. Research Interests
  4. Computational Intelligence
  5. Uncertainty and generalized information theory

Uncertainty and generalized information theory

From the theoretical foundations of computational intelligence in cognitive science and artificial life, a key problem is how information, symbols, representations and the like can arise from a purely dynamical system of many components. Similarly, the study of how information and technology enable collective intelligence, including the collective organization of societies, is of theoretical importance for computational social science. We have contributed to the study of such problems with both theoretical and applied projects. On the theoretical side, we have developed the concepts of selected self-organization, biosemiotics, and material representations. On the applied front, we are interested in using design principles from nature, particularly from biological systems dealing with information and memory, to improve information technology and to study collective intelligence. Additionally, we have used statistical prediction [Kolchinsky and Rocha, 2011] and developed information theoretical methods to study multi-scale modularity and inference in the dynamics of complex brain and biological networks [Kolchinsky et al 2014; Kolchinsky, Gates and Rocha, 2015; Correia, Navarro-Costa and Rocha, 2020; Correia et al, 2022; Parmer, Rocha, & Radicchi, 2022].

We have also worked on mathematical models of uncertainty such as Fuzzy Set Theory and the Dempster-Shafer Theory of Evidence (DST). In particular, We developed a set structure named Evidence Sets, which extended Fuzzy Sets with the DST. Evidence sets were developed to address the shortcomings of fuzzy sets as models of linguistic/cognitive categories previously discussed by George Lakoff by providing a set structure capable of dealing better with the contextual nature of cognitive categories while preserving their prototypical effects as observed by Eleanor Rosch. To make evidence sets useful, we developed new measures of uncertainty for continuous domains, since, in their membership degrees, they capture three distinct types of uncertainty: fuzziness, nonspecificity and conflict. I have also used evidence sets and their measures of uncertainty to develop soft computing agents for a digital library and web tool named TalkMine, which is capable of adapting to different user personalities and learning new terms for existing documents. More information about evidence sets is available in a separate page. The figure depicts a non-consonant evidence set.

Evidence Sets

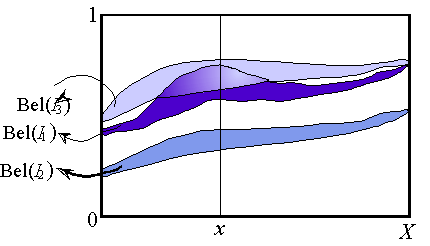
Non-Consonant Evidence Set. The membership degree of an element in a set is defined by a set function known as a basic probability assignment. See details in [Rocha, 1999]



Project Members (Current and Former)

Luis Rocha (PI)

Alaa Abi-Haidar

Rion Brattig Correia

Manlio DeDomenico

Alex Gates

Artemy Kolchinsky

Ana Maguitman

Filippo Radicchi

Jordan C. Rozum

Olaf Sporns

Thomas Parmer



Selected Project Publications